
Welcome
Introduction
Links and Refs
Standard Model
Accelerators
Relativity
Nobel Prizes
String Theory
Unification
Extra dimensions
Sociology
Duality
Internet
Branes
Black holes
M-Theory
Reactions
Mail me
II. The Standard Model
In the previous chapter we made the reader familiar with our methodology and goals. In this chapter we introduce the reader to the first physical theory that we need to introduce String Theory, namely the Standard Model. In our introduction, we incorporate a short intuitive explanation of quantum mechanics, and of special relativity. We go on to combine the two to describe the theory of the smallest particles hitherto observed in laboratories.
The small
The most impressive physical theory that has been verified in experiment is without a doubt the Standard Model. The adjective "Standard" is one of reverence, and refers to the fact that it sets the standard for new attempts at explaining nature. The Standard Model is incredibly accurate. Moreover, the whole name indicates that it is a choice of model amongst many -- not our choice, but the one chosen by nature. What were the models nature could choose from to describe the interactions between tiny particles, and which one did it choose ? That's one of the questions we address in this chapter.
Quantum Mechanics
To explain the limitations on the choice of theory, we need to explain two necessary ingredients that are by now part of the folklore of physics known to most laymen. The first ingredient is quantum mechanics. It is not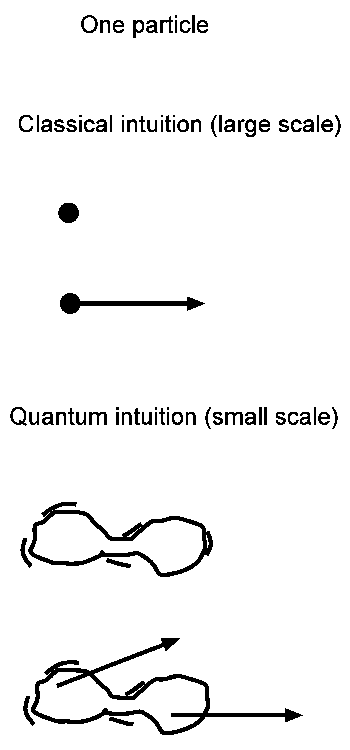 the easiest physical theory to understand. The basic reason is that tiny
bits of matter do not behave in the same way as big chunks of matter. Only
with the behavior of the latter are we familiar in our everyday lives. We
have a fairly good intuition for how a chair in the kitchen behaves, or
a bicycle on the road. This behavior of big chunks of matter (objects) is
well described by classical mechanics. We have a good intuition for the
theory of classical mechanics from our everyday experience.
the easiest physical theory to understand. The basic reason is that tiny
bits of matter do not behave in the same way as big chunks of matter. Only
with the behavior of the latter are we familiar in our everyday lives. We
have a fairly good intuition for how a chair in the kitchen behaves, or
a bicycle on the road. This behavior of big chunks of matter (objects) is
well described by classical mechanics. We have a good intuition for the
theory of classical mechanics from our everyday experience.
Unless we are atomic physicists or high-tech electronic engineers, most of us are hardly familiar with the behavior of tiny bits of matter. We never see individual atoms, or molecules in our everyday lives. As a consequence, we have no good intuition for how they behave. Therefore, most of us have a bad intuition for the theory of quantum mechanics that describes the behavior of individual atoms, or even smaller bits of matter. That's why it has the reputation to be a difficult theory.
But it should be clear now that if we let go of our intuition for how big objects behave, and just trust what highly accurate experiments tell us about the behavior of tiny bits of matter, we might build a new kind of intuition, for how atoms behave. If we are prepared to do that, quantum mechanics will become easier to understand. We'll have an everyday, classical intuition for big objects, and a trained, quantum mechanical intuition for small particles. Let's set out to give you some intuition for how tiny particles behave.
Basic intuition
 To build a basic intuition
for the theory of quantum mechanics, it is sufficient to acquaint ourselves
with a few phenomena that are generic to ultra small particles. One of
those phenomena is the following. Atoms (and other tiny bits of matter,
like molecules, or electrons, etcetera) turn out not to be able to sit
perfectly still. They are always jittering just a tiny little bit. When
we would try to do keep them perfectly still, we would fail. The particle
would overpower our grip and escape very fast to another place. That statement
is the basic content of one of Heisenberg's uncertainty principles. When
we try to fix the position of a particle, it develops a high uncertainty
in momentum ('it escapes'). Vice versa, when we try to measure the momentum
of a particle very accurately (instead of its position), we won't be able
to tell exactly where it is located. The particle will become more like
a cloud then a particle. The first basic intuition for quantum mechanics
that we will use in the following: particles are not point like objects
with fixed velocity, but should be thought of more as (tiny) clouds that
jitter and move. Note that this notion is not difficult to grasp. It just
goes against our classical intuition, that's all. But since we abandoned
that intuition at the end of the previous paragraph, we have no difficulty
incorporating this first basic intuition for quantum mechanical physics.
(Let's call this bit of intuition QM1.)
To build a basic intuition
for the theory of quantum mechanics, it is sufficient to acquaint ourselves
with a few phenomena that are generic to ultra small particles. One of
those phenomena is the following. Atoms (and other tiny bits of matter,
like molecules, or electrons, etcetera) turn out not to be able to sit
perfectly still. They are always jittering just a tiny little bit. When
we would try to do keep them perfectly still, we would fail. The particle
would overpower our grip and escape very fast to another place. That statement
is the basic content of one of Heisenberg's uncertainty principles. When
we try to fix the position of a particle, it develops a high uncertainty
in momentum ('it escapes'). Vice versa, when we try to measure the momentum
of a particle very accurately (instead of its position), we won't be able
to tell exactly where it is located. The particle will become more like
a cloud then a particle. The first basic intuition for quantum mechanics
that we will use in the following: particles are not point like objects
with fixed velocity, but should be thought of more as (tiny) clouds that
jitter and move. Note that this notion is not difficult to grasp. It just
goes against our classical intuition, that's all. But since we abandoned
that intuition at the end of the previous paragraph, we have no difficulty
incorporating this first basic intuition for quantum mechanical physics.
(Let's call this bit of intuition QM1.)
A second basic intuition departs from classical intuition even more drastically. Classically, one would tend to believe that electrons are point particles, and that there is no sense in which they can rotate around an axis. But when looked at from close by, they turn out to do something extremely similar to rotating around an axis. More peculiarly, when they rotate around a specific axis, they can only rotate at a certain rate clockwise or at the same rate counterclockwise. They cannot rotate at any other speed then a given number. In other words, we can specify their rotation by saying clockwise (or 'spin up') or counterclockwise (or 'spin down'). That specifies completely the rotation state they are in. (We don't need to specify how fast they rotate, since the speed of the rotation is fixed.) Electrons therefore have a finite (or 'discrete') number of different states they can be in. To be more precise, electrons generically can be in a state that can be described as a linear combination of two states. Classically they have no such property.
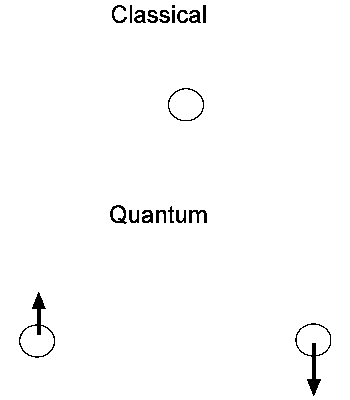 This property too is generic
for small particles. All of them can be in (linear combinations of) one,
two, three, four or five different states. When they can be in one state,
we say they have spin zero, when they can be in two, we say they have
spin 1/2, when they can be in three, they have spin 1, when they can be
in five, they have spin 2. In the course of this book, we will meet particles
of all kinds of spin, from zero to two -- the experts may notice that
certain peculiar theories incorporate particles with even higher spins
--. Particles with integer spin (0, 1 or 2) we will call bosons, and particles
with spin (1/2 or 3/2) we call fermions. We distinguish the two groups
because they differ drastically in their behavior. We return to their
properties later. For now, we note from the above that electrons have
spin 1/2, and that they are fermions. We have now developed a new intuition
for small particles that is also part of the basic properties of quantum
mechanics. Small particles carry spin. We need to specify their spin to
know at how many different velocities they can spin around their own axis.
(Let's call this principle QM2)
This property too is generic
for small particles. All of them can be in (linear combinations of) one,
two, three, four or five different states. When they can be in one state,
we say they have spin zero, when they can be in two, we say they have
spin 1/2, when they can be in three, they have spin 1, when they can be
in five, they have spin 2. In the course of this book, we will meet particles
of all kinds of spin, from zero to two -- the experts may notice that
certain peculiar theories incorporate particles with even higher spins
--. Particles with integer spin (0, 1 or 2) we will call bosons, and particles
with spin (1/2 or 3/2) we call fermions. We distinguish the two groups
because they differ drastically in their behavior. We return to their
properties later. For now, we note from the above that electrons have
spin 1/2, and that they are fermions. We have now developed a new intuition
for small particles that is also part of the basic properties of quantum
mechanics. Small particles carry spin. We need to specify their spin to
know at how many different velocities they can spin around their own axis.
(Let's call this principle QM2)
Special Relativity
Up till now we discussed (two basic features of) the first ingredient that we needed to explain the limitations on the set of models nature could chose the Standard Model from. The model has to incorporate quantum mechanics. The second limitation on a choice of model is set by the theory of special relativity. We need to develop a similar intuition for special relativity as we did for quantum mechanics.Quantum mechanical effects typically become important when tiny particles are involved. Special relativity effects come into play when objects move very fast. Similarly as before, special relativity is not too difficult to understand once we realize that we need to abandon our classical intuition for velocity when objects move at speeds close to the speed of light. Once we abandon those notions, we can easily grasp some of the salient features described by special relativity.
The basic principle that underlies special relativity is that no object can travel faster than the speed of light (SR1). That is an easy enough statement to understand, although it seems very counterintuitive. One example where our intuition fails is the following. When a light ray moves to the right at the speed of light, and we measure how fast it travels, we measure the speed of light. Another experimentator on a train that moves at any velocity to the left relative to us, who measures the speed of the same light ray, will find exactly the same value as we did. (Newton would have expected him the find a velocity which is the velocity of the train plus the velocity we measured. By our first basic principle, that is impossible because that would mean that there is a ray that travels faster than the speed of light.) Although there are many paradoxical and counterintuitive aspects to that basic principle of special relativity, in practice it turns out to be true to the very high degree of accuracy obtained in experiment. Contrary to what many newspaper articles seem to suggest, not a single violation of that basic principle has been observed. Since the basic principle is easy to understand and we will not need its intricate consequences in the following, we won't go into the theory in more detail. It is sufficient to realize that the verified theory of special relativity puts a second severe experimental constraint on the models we had to consider to find the model Nature chose.
Quantum field theory
Both these modifications to classical mechanics, namely the theory of quantum mechanics which describes small objects like atoms and electrons, and the theory of special relativity, which predicts the behavior of slow and fast moving objects, had to be combined in one physical theory. The theory that achieves this goal is highly restricted. The general name for that theory is quantum field theory (or QFT). It is already clear from the previous explanations of the principles underlying quantum mechanics and special relativity what the basic principles that underlie quantum field theory are. On the one hand, quantum fields incorporate the possibility of the fuzziness
in positions and speed of electrons (or atoms) (QM1), and a quantum field
can consist of several component fields that describe the different discrete
states a particles can be in (QM2). The electron quantum field, for instance,
has two components, since the electron has spin 1/2.  The name quantum field derives from the fuzziness of particle positions
in quantum mechanics. Indeed, particles are better thought off as clouds,
and such clouds are better represented by a field. A field should in this
context be thought of as a mathematical way to represent something that
is spread out in space. It describes how a particle is spread out in space.
Moreover, particles described by quantum fields cannot travel faster than
the speed of light. That we know from special relativity (SR1).
The name quantum field derives from the fuzziness of particle positions
in quantum mechanics. Indeed, particles are better thought off as clouds,
and such clouds are better represented by a field. A field should in this
context be thought of as a mathematical way to represent something that
is spread out in space. It describes how a particle is spread out in space.
Moreover, particles described by quantum fields cannot travel faster than
the speed of light. That we know from special relativity (SR1).
We have come a far way already in understanding the nature of the Standard Model. Our previous knowledge of nature taught us that the Standard Model has to be a quantum field theory. That gives us a great deal of knowledge about the Standard Model, in particular, this statement teaches us many of the basic principles the Standard Model is based on. But it doesn't tell us everything. Note that our theoretical reasoning in the last paragraphs, built on underlying principles of accumulation and unification already taught us a lot. But we need experimental input to fix which quantum field theory we can call the Standard Model. It turns out there are many quantum field theories. So the question theoretical physicists tried to solve starting in the forties was: which quantum field theory actually describes nature best ?
Big experiments
From our preliminary remarks it should be easy to derive an arena in which to test which quantum field theory nature chose. Indeed, we can try to build an experiment in which small objects travel at speeds close to the speed of light, and then quantum mechanics and special relativity both become relevant corrections to classical mechanics. In those circumstances, we need the unified theory of quantum fields to understand the things we see. And we can then check which quantum field theory predicts the phenomena we observe.It is not easy to isolate tiny objects, like atoms, or even better, electrons, neutrons or protons, and to accelerate them to high speed. There are only a few places on the planet where thousands of people have collaborated to build tunnels of kilometers length in which they constructed so called particle accelerators. In these particle accelerators, electrons and protons are accelerated to speeds extremely close to the speed of light, and are then made to collide with each other. This creates spectacular events, that happen very fast, and that are very cumbersome to photograph and analyze. The latest technology in electronics, computer science, magnets, superconducting metals, etcetera is needed to make the construction of those machines possible. Indeed, many a technological innovation was made by the engineers and scientists that tried to make their huge particle accelerators work in new and creative ways.
These big accelerators are extremely intriguing machines, and the people that operate them are modern day heroes, showing an amazing ability to cooperate, operate and analyze in a high-tech environment. Some features of these big experiments, and some particular set-ups across the globe are highlighted in the intermezzo following this chapter.
What quantum field theory predicts what happens at the spectacular events in these humongous particle accelerators ? What particles are observed in these events ? Are they the particles we know already, and are the forces with which they interact the ones we are familiar with in our daily lives ? The full answer to these questions is given by the Standard model.
The Standard Model
The Standard Model is a quantum field theory. To specify the quantum field theory, we need to make precise what the elementary building blocks of nature are, and how they interact with each other. For each elementary building block, we will introduce a quantum field, and for every force acting between particles too, we will introduce a quantum field.A basic example of specifying elementary building blocks and their interactions is to say: nature is made of charged particles, and like charges repel each other. and opposite charges attract. The Standard Model makes similar but slightly more complicated statements. We will first specify one third of the elementary building blocks in the Standard Model, then the forces between them, and then we will see how the other two thirds of the building blocks behave in an entirely similar manner as the first third of the building blocks.
The first family
A first family of particles that appears in the tables of the Standard Model is almost entirely familiar. We find the electron, and the up- and down-quark. The electron is familiar as the particle that circles atomic nuclei. Moreover, three
| Name | spin | Interactions | Electric charge |
| Up-quark ('u') | 1/2 | em, weak, strong | +2/3 |
| Down-quark ('d') | 1/2 | em, weak, strong | -1/3 |
| Electron ('e') | 1/2 | em, weak | -1 |
| Electron-neutrino ('nu_e') | 1/2 | weak | 0 |
| The table of the first family of particles in the Standard Model | |||
of these quarks can make up a proton (up,up,down) or a neutron (down,down,up), who in their turn make up the atomic nuclei themselves. All of these particles are therefore more or less familiar. To complete the first family of particles, we also tabled the electron-neutrino that appears, for instance, when unstable atomic nuclei decay into other atomic nuclei. Then they can emit an electrically neutral particle called the electron-neutrino. These four kinds of particles carry spin 1/2. The first family of elementary building blocks is simple to describe, and yet all the matter that we are acquainted with is made up out of these four elementary constituents. In particular, for instance all chemical elements (i.e. the whole table of Mendeljev) can be built out of these four elementary building blocks. And yet, the first family of elementary constituents does not tell the whole story.
The messengers
An important second ingredient are the messengers of forces. Indeed, forces between elementary particles are transmitted through other particles.
| Name | Spin | Messenger for |
| Photon ('gamma') | 1 | The Electromagnetic force |
| Vector boson, ('W,Z') | 1 | The Weak force |
| Gluon, ('A') | 1 | The Strong force |
| The table of messenger particles in the Standard Model. | ||
There are three kinds of messenger particles. There is one very familiar one, the photon, that transmits the electromagnetic force, and that we recognize in our daily lives as ordinary light, radio waves, microwaves, UV-radiation, roentgen radiation, etcetera. All these phenomena result from the first messenger particle.
The second kind of messenger is the weak force transmitter. It is called the vector boson. The weak force, since it is weak, does not affect our daily lives in too high degree, but it is a force present at the atomic scale. And then there is the messenger for the strong force, called gluon. It is the strong force that overpowers the electromagnetic repulsive force between protons, to keep atoms from blowing up.
Interactions
The way the messengers interact with the particles, and therefore how the particles interact with each other., is complicated and is most easily explained in the mathematical language called group theory (and furthermore, by the mathematics of fibre bundles), which we will not go into. However, we can make a list of the particles, and to which forces they are susceptible, and we can already learn a lot of things from looking at the list and accepting a few facts about these interactions that we can learn from elementary reasoning, and daily experience.The photon is the messenger for the electromagnetic interactions, and we already know that it is the charges of the particles that determine whether they interact or not. The photon therefore interacts with charged particles, and its net effect is to make like charged particles repel, and oppositely charged particles attract.
Next, consider protons in an atomic nucleus. The proton is made up of two up-quarks (u) and one down quark (d), yielding a total electric charge (2x2/3)-(1x1/3)=+1. Thus, all protons have positive charge +1 and therefore they are repelled by the electromagnetic force. Nevertheless, they stay together. In other words, there must be a stronger force than the electromagnetic force that keeps protons glued together in the atomic nucleus. Indeed, it is the gluons, responsible for the strong force that keep protons together in an atomic nucleus. And, if we check our table, we indeed see that the quarks that make up protons interact strongly, so this explanation is credible.
Note also that the electron-neutrino (nu_e) interacts only via the weak force. Therefore it is very difficult to observe directly. It tends to fly through all kinds of matter, since it does not feel the electromagnetic force, nor the strong force. It can travel for vast distances without being disturbed by any of the surroundings. Actually, neutrinos are flying through your body right now, without you noticing. (Indeed, a bullet that 'hits' you interacts with your body (almost) only with the electromagnetic force, via its protons and electrons. That's why a neutrino doesn't 'hit' you like a bullet does -- it does not interact electromagnetically..)
Almost all of the natural phenomena that we see around us can be described in terms of these kinds of elementary particles. Substances are made of molecules, which in turn are made of atoms. Atoms are made of electrons, protons and neutrons. Protons and neutrons are made of up and down quarks. The nucleus of an atom is kept together by the strong force. And photons, light, is all around us. That describes fairly accurately the content of our kitchen, our body, the earth, etcetera. But physicists found a lot more elementary particles than were needed at first sight.
The second and third family
Nature copied and pasted. It turns out that there is not one family of elementary matter particles, but three. And the second and the third family look exactly like the first family. The new matter particles can be described as follows (-- the reader is invited to compare these tables to the previous one --):
| Name | spin | Interactions | Electric charge |
| Charm-quark ('c') | 1/2 | em, weak, strong | +2/3 |
| Strange-quark ('s') | 1/2 | em, weak, strong | -1/3 |
| Muon ('mu') | 1/2 | em, weak | -1 |
| Muon-neutrino ('nu_mu') | 1/2 | weak | 0 |
| The table of the second family of particles in the Standard Model | |||
| Name | spin | Interactions | Electric charge |
| Top-quark ('t') | 1/2 | em, weak, strong | +2/3 |
| Bottom-quark ('b') | 1/2 | em, weak, strong | -1/3 |
| Tauon ('tau') | 1/2 | em, weak | -1 |
| Tauon-neutrino ('nu_tau') | 1/2 | weak | 0 |
| The table of the third family of particles in the Standard Model | |||
Note that these are just copies of the first table of elementary particles. And we therefore have a good understanding already of how these new particles interact amongst each other. Indeed, they interact in the same way as the previous family that we discussed, with the same (!) messenger particles. There aren't any other messenger particles than the ones we had before. That fact makes us suspect that perhaps the new families also interact with the old family, and with each other., since they all interact with the same messengers. And indeed they do. As an example, the s-quark attracts the d-quark electromagnetically.
If you understood all of the explanations above, you understand that
there are now zillions of possible interactions amongst all the elementary
particles. So, to get an understanding of what's going on, it won't help
to enumerate all of them. We should develop some intuition for what is
possible, and what is impossible. 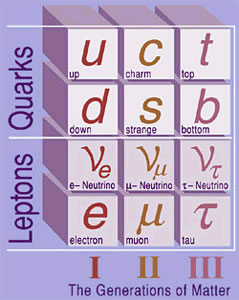
One to Three
Why is it, first of all, that we are all familiar with the matter built up out of the first family, and not at all with the matter built up from the second and third family ? The answer is simple. It is because the second and third family are much heavier than the first. (For example, the strange quark is approximately twenty times heavier than its cousin, the down quark.) And since mass is energy, we need much more energy to build matter out of the second and third family. Moreover, once we built such a system, it would tend to decay to a system built out of the first family (very rapidly). Systems built out of the first family do not decay further because of conservation laws.Next to the energetics of particles, there is indeed a second basic principle that governs the interactions of particles. There are many laws that say which interaction processes are possible, and which are not. One conservation law is that electric charge is conserved in all elementary processes (and therefore also in all macroscopic processes). When a proton and a proton interact, they can only produce a bunch of particles with total electric charge +2, because electric charge is conserved. An electron, which is the lightest negatively electrically charged particle can therefore not decay. When we combine the energetics with all possible conservation laws, we learn a lot about why our world is stable, and is only made up of the first family of elementary particles.
To repeat, we need a lot of energy to make the particles in the second
and third family (when we ignore the almost massless neutrinos). They
are only made in extreme circumstances, as in particle accelerator experiments
(or the interior of stars, or the big bang under circumstance of extremely
high pressure or heat). In accelerators they appear as a result of the
collision of two high energy bundles of elementary particles of the first
family. Then, very soon after, they decay again into members of the first
family, and messengers. The reason is that there is nothing to stop anti-matter from annihilating with matter very rapidly.
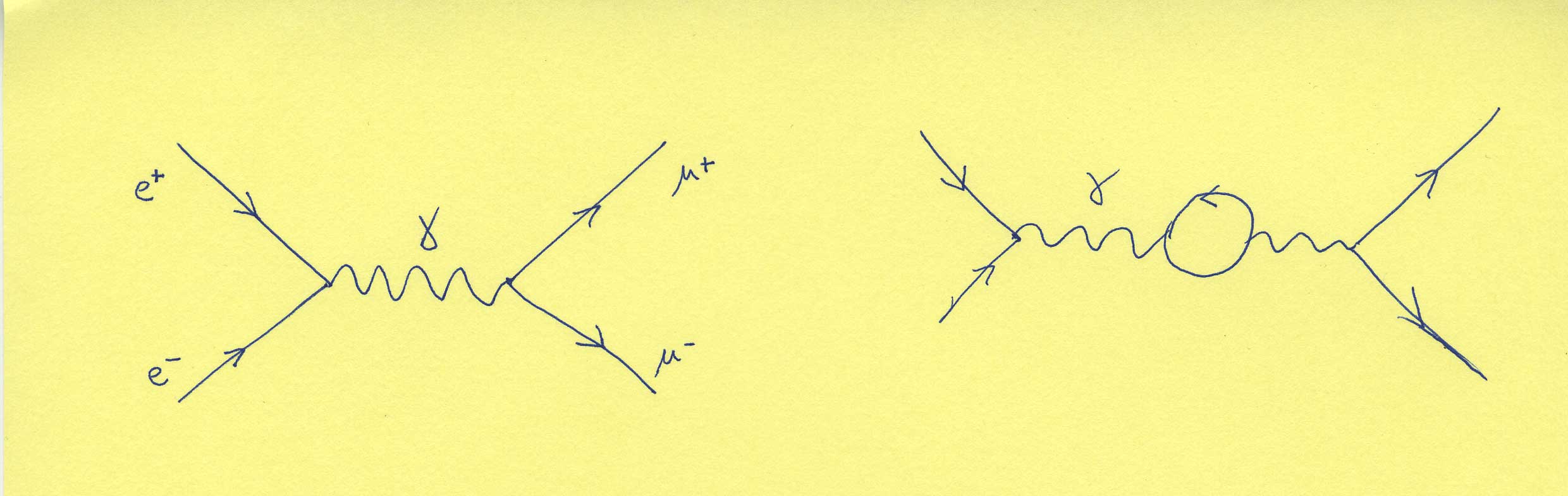
An electron and positron (i.e. anti-electron) have opposite charge, and can annihilate very easily into
neutraly charged photons. An anti-car would very rapidly annihilate with the street beneath it, and the air
around it. The world is made of matter, and is stable, because there is more matter around than there is anti-matter.
In other words, there is no further anti-matter around for the matter that is present to annihilate with. That's one of
the reasons why an (ordinary matter) car is fairly stable. It's surrounded by other matter with which it cannot annihilate,
due to conservation laws. 
Anti-matter
All particles with spin 1/2 have an almost identical counterpart, with the same mass,
and with exactly opposite charge. For instance the electron has a positron counterpart.
(A more standardized name for the positron would be anti-electron.) These counterparts
make up anti-matter. For instance, we can make anti-hydrogen from two anti-up quarks,
one anti-down quark, and one anti-electron (or positron). In anti-hydrogen, we have a positive
charge positron cloud surrounding a negatively charged nucleus (which is an anti-proton). The properties
of anti-hydrogen are (very nearly) identical to those of hydrogen. Except that it consists of anti-matter instead
of matter. So, why don't we see anti-cars around ?